
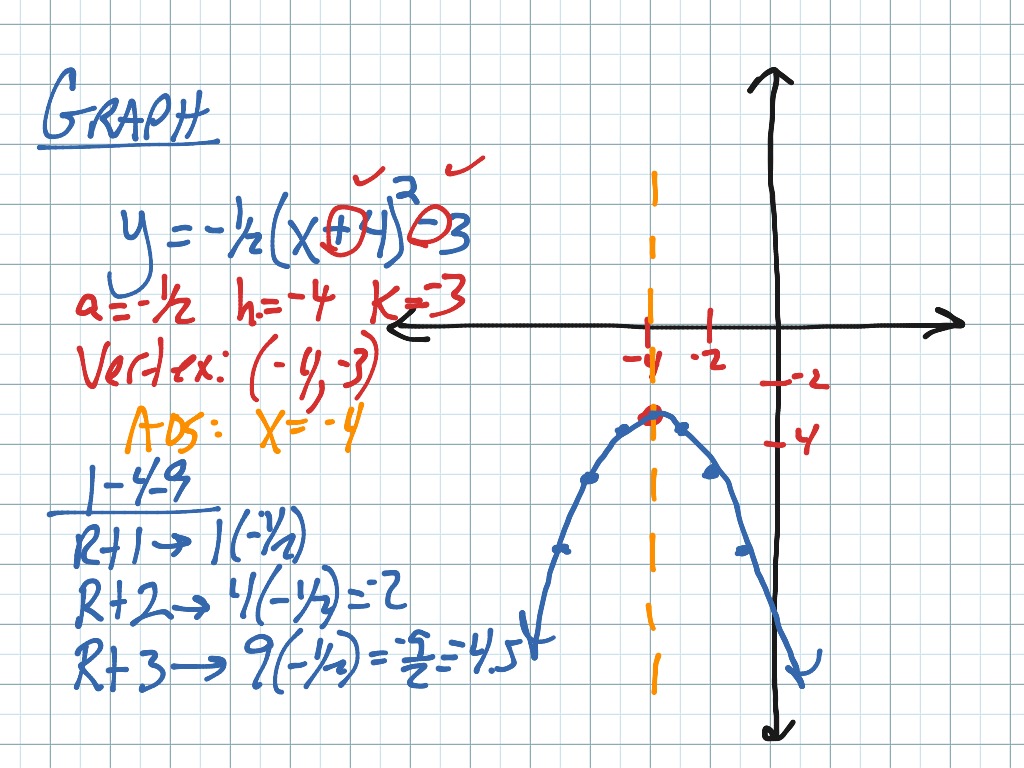
The output of the quadratic function at the vertex is the maximum or minimum value of the function, depending on the orientation of the parabola. Since the leading coefficient, 2, is positive, this means the parabola opens upward, and thus has a minimum value.Ī.) In vertex form, f(x) = 2(x - 2)² + 1 and therefore has a minimum value of 1. The standard form of a quadratic function presents the function in the form f(x)a(xh)2+k where ((h, k)) is the vertex. There are many reasons: from a geometric point of view, the vertex form allows use to see the given quadratic function as a translation and rescaling of an elementary parabola, where there translation is determined by the vertex, and the scale is determine with the leading coefficient. In a parabola, which is the graph of a quadratic function, the y-coordinate of the vertex is either the minimum or maximum value. A parabola is the graph of a quadratic equation, which is an equation that has its variable, usually x, raised to. Then, substitute the vertex into the vertex form equation, ya(x-h)2+k.
#VERTEX FORM OF A QUADRATIC FUNCTION HOW TO#
The x-coordinate of the vertex is x = -b / 2a Now that we have values for a, h, and k, we can write a general equation for our parabola using vertex form Remember, vertex form is ya(x-h)2+k. How to Convert Standard Form to Vertex Form. Finding the vertex of the quadratic by using the equation x-b/2a, and then substituting that answer for y in the orginal equation.
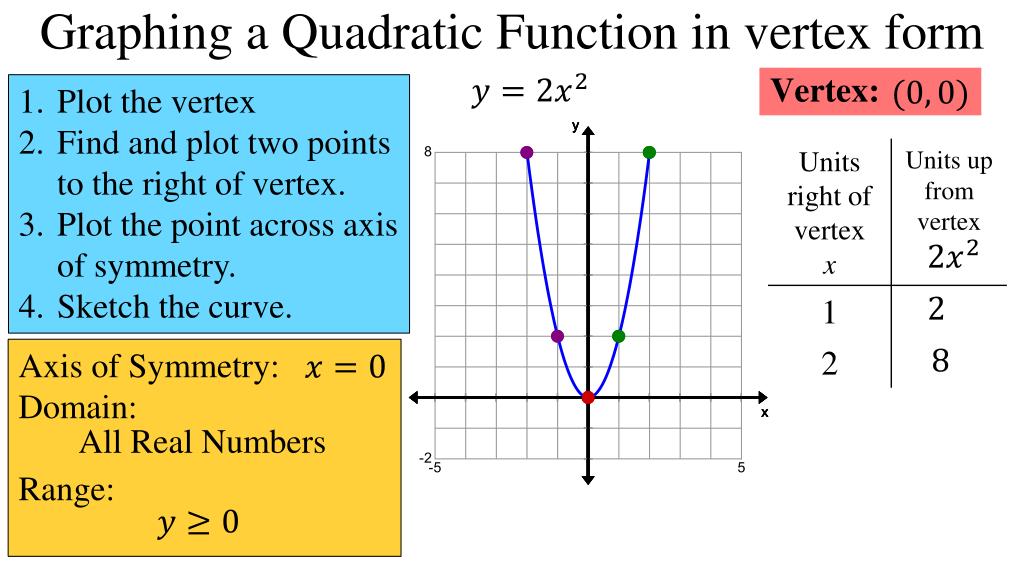
To begin, find the x and y coordinates of the vertex, then substitute them along with a which is 2, into vertex form. To find the vertex of a quadratic equation, y ax2 + bx + c, we find the point (- b / 2 a, a (- b / 2 a) 2 + b (- b / 2 a) + c ), by following these steps. The equation f(x) = 2x² - 8x + 9 is in standard form and must be transformed into vertex form.


 0 kommentar(er)
0 kommentar(er)
